Conical Compression Springs

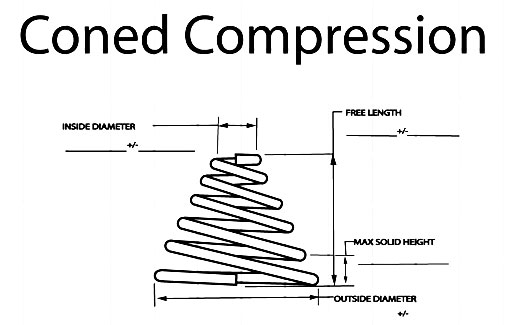
Whether you are developing a conical compression spring design or simply ordering your springs, it is important that you provide the following spring specifications and information.

• Free Length, Maximum, Minimum.

• Controlling Diameter, Outside Diameter Maximum. Inside Diameter Minimum. Pitch Diameter. Works Inside (Dia. Hole). Works Over (Dia. Shaft).

• Number of Coils.

• Wire Size. Decimal size if possible. Material, Kind and Grade.

• Loads at deflected positions.

• Style of Ends, (see illustrations). Right or Left Hand Wound.

• Finish. Plain unless otherwise specified.

• Maximum Solid Length.

• Frequency of Compression.

How to Determine Rate For Conical Compression Springs

Conical Compression change in load per unit deflection, may be determined by the following procedure:

1. Deflect spring to approximately 20 percent of available deflection and measure load (P1) and spring length (L1).

2. Deflect spring to approximately 80 percent of available deflection and measure load (P2) and spring length (L2). Be certain that no coils (other than closed ends) are touching L2.

3. Calculate rate (R) lb./in. (N/mm)
   R = (P2 - P1) / (L1 - L2)

    

Solid Height

The solid height of conical compression springs are defined as the length of the spring when under sufficient load to bring all coils into contact with the adjacent coils and additional load causes not further deflection.   Solid height should be specified by the user as a maximum, with the actual number of coils in the spring to be determined by the spring manufacturer.

As square or rectangular wire is coiled, the wire cross-section deforms slightly into a keystone or trapezoidal shape, which increased the solid height considerably.  The dimensional change is a function of the spring index and the thickness of the material.

 

Conical Compression Pitch

A uniform pitch in a conical spring is far easier to make then a variable pitch. During testing, if no coil bottoms, a straight-line force curve versus deflection occurs similar to that of a compression spring. When the largest active coil bottoms, an upward slope of the force curve occurs. The maximum force occurs when all coils have bottomed. The calculation to determine the force at the solid position can be easily performed by using the regular formula for compression springs and using the mean diameter of the smallest active coil only; N equals 1.

 

Grinding

Grinding the end coils of conical springs is done in a different manner. Some specifications call for grinding only the small end coil. Others require grinding on both ends. Grinding in either case is difficult and expensive. Grinding small, medium and large quantities may be done as explained for regular compression springs except that when using double wheel grinders it is necessary to make special turntables with holes shaped like the conical springs. Making a large amount of tapered holes to hold the springs is not easily done and may require making special coned reamers to cut the holes. For this reason, hardwood platens, in place of steel are often used.

 

Cautions

1. Extra time for all manufacturing operations should be considered when estimating the costs of making coned conical compression springs or for any springs requiring special material, square or rectangular wire, or special testing.

2. Variable pitches in a conical spring are occasionally specified so that all active coils bottom simultaneously, but this is seldom achieved.

3. Grinding is especially difficult and time-consuming. Special fixtures may be required. The cost of grinding discs should be included for large quantities of springs.

4. Tangling of highly pitched springs made from small diameters of wire up to 1/16 inch (1.5 mm) having rather large base diameters often occurs and may require special handling during secondary operations and for packaging.